مبرهنة لاغرانج (نظرية الزمر)
في نظرية الزمر، مبرهنة لاغرانج (بالإنجليزية: Lagrange's theorem) هي مبرهنة تنص على أنه إذا كانت G زمرة منتهية وH زمرة جزئية من G فإن رتبة H (أي عدد العناصر الموجودة فيها) قاسم لرتبة G.[1][2][3] سميت هذه المبرهنة هكذا نسبة إلى عالم الرياضيات جوزيف لاغرانج.
لمعانٍ أخرى، انظر مبرهنة لاغرانج (توضيح).
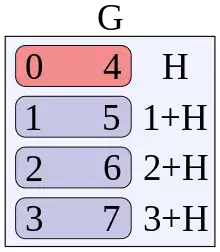
G هي الزمرة , the حسابيات نمطية تحت عملية الجمع. الزمرة الجزئية H تحتوي على العنصرين 0 و4، فقط وis isomorphic to . There are four left cosets of H: H itself, 1+H, 2+H, and 3+H (written using additive notation since this is an زمرة أبيلية). Together they partition the entire group G into equal-size, non-overlapping sets. Thus the index [G: H] is 4.
البرهان على مبرهنة لاغرانج
نعتبر علاقة التكافؤ xRy يكافئ x-y ينتمي إلى H...نبين ان عدد عناصر اصناف H+x لها نفس عدد عناصر H.تم نستنتج ان عدد عناصر الزمرة الجزئيةH يقسم عدد عناصر الزمرةG.وهدا الخارج يسمى مؤشر H.
مراجع
- Lagrange, J. L. (1771). "Suite des réflexions sur la résolution algébrique des équations. Section troisieme. De la résolution des équations du cinquieme degré & des degrés ultérieurs" [Series of reflections on the algebraic solution of equations. Third section. On the solution of equations of the fifth degree & higher degrees]. Nouveaux Mémoires de l’Académie Royale des Sciences et Belles-Lettres de Berlin: 138–254. مؤرشف من الأصل في 26 يناير 2020. الوسيط
|CitationClass=تم تجاهله (مساعدة) - Camille Jordan (1861). "Mémoire sur le numbre des valeurs des fonctions" [Memoir on the number of values of functions]. Journal de l'École Polytechnique. 22: 113–194. مؤرشف من الأصل في 26 فبراير 2018. الوسيط
|CitationClass=تم تجاهله (مساعدة) - pp. 41-50. نسخة محفوظة 15 ديسمبر 2019 على موقع واي باك مشين.
- بوابة رياضيات
- بوابة جبر
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.