صيغة كوشي التكاملية

${\displaystyle f(a)={1 \over 2\pi i}\oint _{C}{f(z) \over z-a}\,dz\!}$

ومن هذه الصيغة يمكن استنتاج قابلية هذا التابع للمفاضلة بعدد لا نهائي من المرات

${\displaystyle f^{(n)}(a)={n! \over 2\pi i}\oint _{C}{f(z) \over (z-a)^{n+1}}\,dz.}$

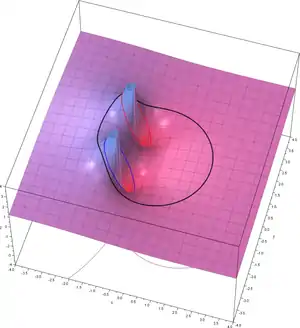
المساحة (أو السطح) الممثلة للجزء الحقيقي للدالة g(z) = z² / (z² + 2z + 2) and its singularities, with the contours الموصوفة في النص.

لتكن الدالة

${\displaystyle g(z)={\frac {z^{2}}{z^{2}+2z+2}}}$,

• معادلات كوشي-ريمان

• بوابة رياضيات
• بوابة تحليل رياضي