تكامل لوبيغ

في الرياضيات ، يعتبر التكامل لدالة غير سلبية لمتغير واحد في أبسط الحالات كالمساحة بين الرسم البياني لتلك الدالة والمحور x .[1][2][3] يمتد تكامل لوبيغ إلى الدوال من الدرجات الأعلى. كما أنه يوسع المجالات التي يمكن تعريف هذه الدوال عليها.

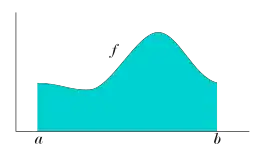
هذه مقالة غير مراجعة. ينبغي أن يزال هذا القالب بعد أن يراجعها محرر مغاير للذي أنشأها؛ إذا لزم الأمر فيجب أن توسم المقالة بقوالب الصيانة المناسبة. يمكن أيضاً تقديم طلب لمراجعة المقالة في الصفحة المُخصصة لذلك. (يوليو 2020)
يمكن تفسير تكامل دالة إيجابية كمساحة تحت منحنى.

تفسير بديهي
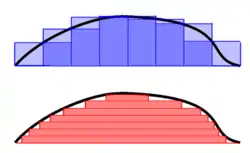
تكامل ريمان داربوكس (باللون الأزرق) وتكامل لوبيغ (باللون الأحمر).

لفهم الأساليب المختلفة للتكامل ، دعنا نتخيل أننا نريد أن نجد حجم جبل (فوق مستوى سطح البحر).

نهج ريمان داربوكس
بإستخدام هذه الطريقة للتكامل نقوم بتقسيم قاعدة الجبل إلى شبكة من المربعات مساحتها متر واحد. ثم نقوم بقياس ارتفاع الجبل في وسط كل مربع. الحجم مقسوماً على مربع شبكة واحد يساوي تقريباً 1متر مربع × (ارتفاع ذلك المربع) ، لذا فإن الحجم الإجمالي هو 1م 2 مضروباً في مجموع الارتفاعات.
نهج لوبيغ
ارسم خريطة خطوط منسوبة للجبل بحيث يبلغ ارتفاع الخطوط المجاورة 1 متر لكل منها. يبلغ حجم الأرض التي يحتويها شكل الخط المنسوب واحد حوالي 1م × (مساحة الشكل) ، لذا فإن الحجم الإجمالي هو مجموع هذه المناطق مضروبة في 1م.
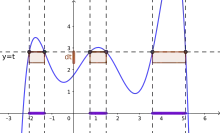
في الصورة دالة قابلة للقياس مع المجموعة (على المحور السيني). يتم الحصول على تكامل لوبيغ عن طريق تقطيع شرائح على امتداد المحور ص ، باستخدام مقياس لوبيغ أحادي البعد لقياس "عرض" الشرائح.

ملاحظات
  1. "معلومات عن تكامل لوبيغ على موقع babelnet.org". babelnet.org. مؤرشف من الأصل في 28 أكتوبر 2020. الوسيط |CitationClass= تم تجاهله (مساعدة)
  2. "معلومات عن تكامل لوبيغ على موقع academic.microsoft.com". academic.microsoft.com. مؤرشف من الأصل في 28 أكتوبر 2020. الوسيط |CitationClass= تم تجاهله (مساعدة)
  3. "معلومات عن تكامل لوبيغ على موقع id.ndl.go.jp". id.ndl.go.jp. مؤرشف من الأصل في 5 مارس 2016. الوسيط |CitationClass= تم تجاهله (مساعدة)

    المراجع
    • Bartle, Robert G. (1995). The elements of integration and Lebesgue measure. New York: John Wiley & Sons Inc. xii+179. ISBN 0-471-04222-6. MR = 1312157 1312157. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Bauer, Heinz (2001). Measure and Integration Theory. Berlin: De Gruyter. 236. ISBN 978-3-11-016719-1. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Bourbaki, Nicolas (2004). Integration. I. Chapters 1–6. Translated from the 1959, 1965 and 1967 French originals by Sterling K. Berberian. Berlin: Springer-Verlag. xvi+472. ISBN 3-540-41129-1. MR = 2018901 2018901. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Dudley, Richard M. (1989). Real analysis and probability. Pacific Grove, CA: Wadsworth & Brooks/Cole Advanced Books & Software. xii+436. ISBN 0-534-10050-3. MR = 0982264 0982264. الوسيط |CitationClass= تم تجاهله (مساعدة) Very thorough treatment, particularly for probabilists with good notes and historical references.
    • Folland, Gerald B. (1999). Real analysis: Modern techniques and their applications. (الطبعة Second). New York: John Wiley & Sons Inc. xvi+386. ISBN 0-471-31716-0. MR = 1681462 1681462. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Halmos, Paul R. (1950). Measure Theory. New York, N. Y.: D. Van Nostrand Company, Inc. صفحات xi+304. MR = 0033869 0033869. الوسيط |CitationClass= تم تجاهله (مساعدة) A classic, though somewhat dated presentation.
    • Hazewinkel, Michiel, المحرر (2001), "Lebesgue integral", Encyclopedia of Mathematics, سبرنجر, ISBN 978-1-55608-010-4 الوسيط |CitationClass= تم تجاهله (مساعدة); الوسيط |separator= تم تجاهله (مساعدة)CS1 maint: ref=harv (link)
    • Lebesgue, Henri (1904). "Leçons sur l'intégration et la recherche des fonctions primitives". Gauthier-Villars. Paris. الوسيط |CitationClass= تم تجاهله (مساعدة)CS1 maint: ref=harv (link)
    • Lebesgue, Henri (1972). Oeuvres scientifiques (en cinq volumes) (باللغة الفرنسية). Geneva: Institut de Mathématiques de l'Université de Genève. صفحة 405. MR = 0389523 0389523. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Lieb, Elliott; Loss, Michael (2001). Analysis. 14 (الطبعة 2nd). جمعية الرياضيات الأمريكية. ISBN 978-0821827833. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Loomis, Lynn H. (1953). An introduction to abstract harmonic analysis. Toronto-New York-London: D. Van Nostrand Company, Inc. صفحات x+190. MR = 0054173 0054173. الوسيط |CitationClass= تم تجاهله (مساعدة) Includes a presentation of the Daniell integral.
    • Munroe, M. E. (1953). Introduction to measure and integration. Cambridge, Mass.: Addison-Wesley Publishing Company Inc. صفحات x+310. MR = 0053186 0053186. الوسيط |CitationClass= تم تجاهله (مساعدة) Good treatment of the theory of outer measures.
    • Royden, H. L. (1988). Real analysis (الطبعة Third). New York: Macmillan Publishing Company. صفحات xx+444. ISBN 0-02-404151-3. MR = 1013117 1013117. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Rudin, Walter (1976). Principles of mathematical analysis. (الطبعة Third). New York: McGraw-Hill Book Co. صفحات x+342. MR = 0385023 0385023. الوسيط |CitationClass= تم تجاهله (مساعدة) Known as Little Rudin, contains the basics of the Lebesgue theory, but does not treat material such as Fubini's theorem.
    • Rudin, Walter (1966). Real and complex analysis. New York: McGraw-Hill Book Co. صفحات xi+412. MR = 0210528 0210528. الوسيط |CitationClass= تم تجاهله (مساعدة) Known as Big Rudin. A complete and careful presentation of the theory. Good presentation of the Riesz extension theorems. However, there is a minor flaw (in the first edition) in the proof of one of the extension theorems, the discovery of which constitutes exercise 21 of Chapter 2.
    • Saks, Stanisław (1937). Theory of the Integral. 7 (الطبعة 2nd). وارسو-لفيف: G.E. Stechert & Co. JFM = complete&q = an:63.0183.05 63.0183.05. Zbl = complete&q = an:0017.30004 0017.30004. الوسيط |CitationClass= تم تجاهله (مساعدة). English translation by Laurence Chisholm Young, with two additional notes by Stefan Banach.
    • Shilov, G. E.; Gurevich, B. L. (1977). Integral, measure and derivative: a unified approach. Translated from the Russian and edited by Richard A. Silverman. New York: Dover Publications Inc. xiv+233. ISBN 0-486-63519-8. MR = 0466463 0466463. الوسيط |CitationClass= تم تجاهله (مساعدة) Emphasizes the Daniell integral.
    • Siegmund-Schultze, Reinhard (2008), "Henri Lebesgue", in Timothy Gowers; June Barrow-Green; Imre Leader (المحررون), Princeton Companion to Mathematics, Princeton University Press الوسيط |CitationClass= تم تجاهله (مساعدة); الوسيط |separator= تم تجاهله (مساعدة)CS1 maint: ref=harv (link).
    • Teschl, Gerald. Topics in Real and Functional Analysis. (lecture notes). مؤرشف من الأصل في 05 نوفمبر 2019. الوسيط |CitationClass= تم تجاهله (مساعدة)
    • Yeh, James (2006). Real Analysis: Theory of Measure and Integral 2nd. Edition Paperback. Singapore: World Scientific Publishing Company Pte. Ltd. صفحة 760. ISBN 978-981-256-6. الوسيط |CitationClass= تم تجاهله (مساعدة)
    تكامل لوبيغ في المشاريع الشقيقة
    • خطأ في استعمال قالب:شريط بوابات: يلزم إعطاء وسيط واحد على الأقل.
    This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.